Coordination Number Of Simple Cubic

Cubic Unit Cell
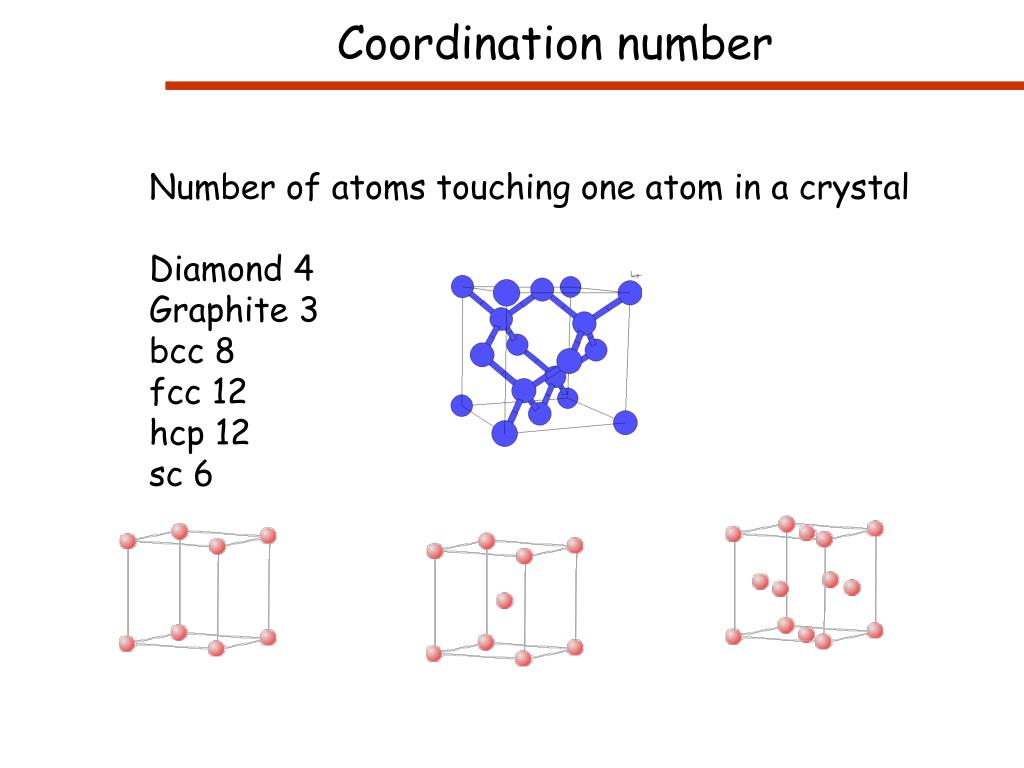
Coordination Number Of Simple Cubic
Coordination Number Body Centered Cubic
Coordination Number Of Simple Cubic System Is
How To Calculate Coordination Number Of Simple Cubic

Return to Class Schedule

In a simple cubic lattice, the co-ordination number is 6 and it contains 1 atom per unit cell. A particular lattice point co-ordinates with 4 lattice points in a plane, 1 above the plane and 1 below the plane i.e. Co-ordinates total 6 lattice points.
Diamond Cubic Coordination Number. Each atom in a diamond cubic crystal is tetrahedrally bonded to 4 other atoms, so there are 4 nearest neighbors (NNs). In other words, the coordination number (CN) is 4. The NN distance is, and the next-nearest neighbor (NNN) distance is, where a is the lattice constant (I’ll prove this below).
In this video I'll explain, What is COORDINATION NUMBER? & How to find coordination number for Simple Cubic, BCC & FCC structures in Tamil.

(5) The coordination number of each lattice point is six. This becomes apparent when inspecting part of an adjacent unit cell. This becomes apparent when inspecting part of an adjacent unit cell. (6) The unit cell contain eight corner spheres, however, the total number of spheres within the unit cell is 1 (only 1/8th of each sphere is actually.

The Structures of Simple Solids

In considering metallic or ionic solids, the atoms and ions are viewed as hard spheres. In metals, packing often results in greatest spatial efficiency, with the metal atoms having lost their valence electrons. The valence electrons are free to move throughout the structure, and result in the high electrical conductivity and high malleability of metallic solids. Ionic solids are viewed as having arrangements in which cations are touching anions, and the anions are touching cations. The crystal is held together by largely coulombic forces of attraction between oppositely charged ions.

Unit Cells and Crystal Structures

Crystals consist of repeating asymmetric units which may be atoms, ions or molecules. The space lattice is the pattern formed by the points that represent the repeating structural units. A unit cell of the crystal is an imaginary parallel sided region from which the entire crystal can be built up. Most crystals have several regions which can serve as a unit cell, so usually, the smallest unit cell which exhibits the greatest symmetry is chosen. If repeated in three dimensions, the entire crystal is recreated.
Metallic solids, lacking directional bonds, often pack with the greatest efficiency, adopting a close-packed structure. Each metal atom has 12 nearest neighbors (CN=12), and 74% of the volume of the unit cell is occupied by the atomic spheres. Close packing can occur in a variety of arrangements. A metal atom, in a plane, will be surrounded by 6 nearest neighbors. Above and below the plane are 'caps' consisting of a triangle of 3 atoms which fits in the recesses formed by the central metal atom and its 6 neighbors. The caps can be aligned with each other (an ABA pattern), or staggered (an ABC pattern). There are also metal crystals which have irregular patterns, and some which repeat after many (several hundred) layers.

The ABA pattern results in the hexagonal close packed unit cell pictured above (a). The ABC pattern results in a face-centered cubic arrangement, also called a cubic close-packed arrangement, as pictured (b). Either structure contains small holes which account for 26% of the crystal volume. There are two types of holes. Octahedral holes, with a coordination number of 6, lie within two staggered triangular planes of atoms. The octahedral holes of a face centered cube (or cubic close packed unit cell) are illustrated below.

The octahedral holes (in orange) are found on the edges and in the center of the unit cell. It's easiest to see the octahedral symmetry of the hole in the center of the cube, as all 6 nearest neighbors are illustrated. For n atoms in a close-packed structure, there are n octahedral holes. The size of an octahedral hole will depend upon the size of the atoms which are close-packed. Ionic solids and alloys are often viewed as a close-packed arrangement of the larger metal or ion, with the smaller atom or ion occupying the holes.
Tetrahedral holes are formed by a planar triangle of atoms with an atom covering the indentation in the center. the resulting hole has a coordination number of 4 in a tetrahedral arrangement. In a face centered cubic unit cell, the tetrahedral holes can be viewed as being behind each corner. The tetrahedral holes are the small lightly colored circles at the corners of the inner cube. There are two types of tetrahedral holes (yellow and white) indicating two different orientations. For a crystal of n atoms in a close-packed arrangement, there are 2n tetrahedral holes.

Cubic Unit Cell

Radii of Octahedral and Tetrahedral Holes

The size of an octahedral hole or a tetrahedral hole in a close-packed arrangement will depend on the size of the ion which is close-packed. Assuming the larger ion (usually the anion) is close-packed, the octahedral holes will have a radius of 0.414 times the radius of the larger ion. The tetrahedral holes will have a radius of 0.225 times the radius of the larger ion. In alloys, both metals are in their elemental form, and their atomic radii must be considered. Atomic radii of metals are obtained in pure metal samples as 1/2 the distance between the nuclei of neighboring metal atoms. However, this distance varies with coordination number, with a coordination number of 12 exhibiting metals with their greatest inter nuclear distance. The following table shows the change in atomic radii with coordination number.

Coordination # relative radius
12 1.00
8 0.97
6 0.96
4 0.88

For comparison of atomic radii, all are corrected, using the above factors, to a coordination number of 12, even if their typical crystal structure has a lower coordination number. These radii are sometimes referred to as Goldschmidt radii.

Ionic Solids

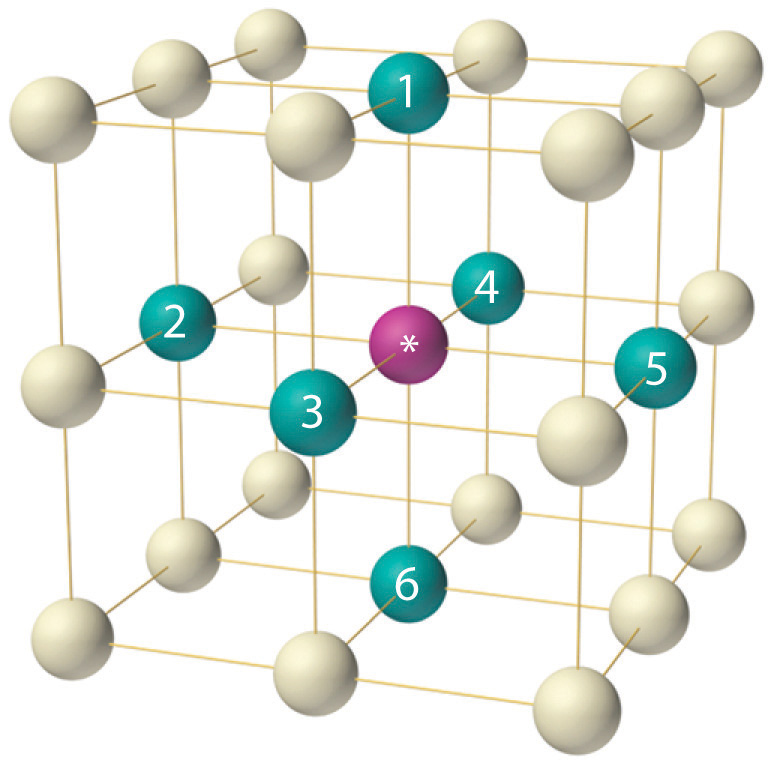
Many ionic structures can be viewed as a close-packed arrangement of the larger ion (usually the anion), with the cations occupying octahedral or tetrahedral holes and distorting the structure so that the anions no longer touch each other. For example, the rock salt structure of NaCl can be viewed as either:

a) as a face centered cubic array of the anions, with the cations occupying the octahedral holes (in the center of each cube and on each edge) or

b) as a face centered array of cations with the anions occupying all the octahedral holes

In either description, each ion has a coordination number of 6, with the unit cell containing an equal number of cations and anions (a 1:1 salt).
Another 1:1 crystal structure for ionic solids is the CsCl structure, with chloride ions in the corners of a cube, and the cesium ion in the center (or cubic hole). The structure can also be viewed in the opposite way, with cesium ions in the corners and chloride in the center. This structure arises in 1:1 salts in which the ions are fairly similar in size. Both ions have a coordination number of 8.
A third structure for 1:1 salts is the zinc-blende or Spalerite structure. The anions are in a face centered array, with cations in half the tetrahedral holes.

Since each ion has four nearest neighbors, the coordination number is 4 in this structure.

Salts with a 1:2 (or 2:1) stoichiometry sometimes exhibit the fluorite (or antifluorite) structures. The fluorite structure, seen in calcium fluoride, has the calcium ions in a face centered cubic array with the fluoride ions in all (8) of the tetrahedral holes. The fluoride ions have a coordination number of 4, and the calcium ions have a coordination number of 8. Another view of this structure is as a simple cubic arrangement of the anions, with the cations occupying half of the cubic holes.

The antifluorite structure is often seen in salts with the general formula of M₂X, such as potassium oxide. The anions are in a face centered cubic arrangement, with the cations in all of the tetrahedral holes.

Return to Class Schedule

Coordination Number Of Simple Cubic

Copyright ©1998 Beverly J. Volicer and Steven F. Tello, UMass Lowell. You may freely edit these pages for use in a non-profit, educational setting. Please include this copyright notice on all pages.

The term 'closest packed structures' refers to the most tightly packed or space-efficient composition of crystal structures (lattices). Imagine an atom in a crystal lattice as a sphere. While cubes may easily be stacked to fill up all empty space, unfilled space will always exist in the packing of spheres. To maximize the efficiency of packing and minimize the volume of unfilled space, the spheres must be arranged as close as possible to each other. These arrangements are called closest packed structures.

The packing of spheres can describe the solid structures of crystals. In a crystal structure, the centers of atoms, ions, or molecules lie on the lattice points. Atoms are assumed to be spherical to explain the bonding and structures of metallic crystals. These spherical particles can be packed into different arrangements. In closest packed structures, the arrangement of the spheres are densely packed in order to take up the greatest amount of space possible.

Types of Holes From Close-Packing of Spheres

When a single layer of spheres is arranged into the shape of a hexagon, gaps are left uncovered. The hole formed between three spheres is called a trigonal hole because it resembles a triangle. In the example below, two out of the the six trigonal holes have been highlighted green.

Once the first layer of spheres is laid down, a second layer may be placed on top of it. The second layer of spheres may be placed to cover the trigonal holes from the first layer. Holes now exist between the first layer (the orange spheres) and the second (the lime spheres), but this time the holes are different. The triangular-shaped hole created over a orange sphere from the first layer is known as a tetrahedral hole. A hole from the second layer that also falls directly over a hole in the first layer is called an octahedral hole.

Closest Pack Crystal Structures

Hexagonal Closest Packed (HCP)

In a hexagonal closest packed structure, the third layer has the same arrangement of spheres as the first layer and covers all the tetrahedral holes. Since the structure repeats itself after every two layers, the stacking for hcp may be described as 'a-b-a-b-a-b.' The atoms in a hexagonal closest packed structure efficiently occupy 74% of space while 26% is empty space.

Cubic Closest Packed (CCP)

The arrangement in a cubic closest packing also efficiently fills up 74% of space. Similar to hexagonal closest packing, the second layer of spheres is placed on to of half of the depressions of the first layer. The third layer is completely different than that first two layers and is stacked in the depressions of the second layer, thus covering all of the octahedral holes. The spheres in the third layer are not in line with those in layer A, and the structure does not repeat until a fourth layer is added. The fourth layer is the same as the first layer, so the arrangement of layers is 'a-b-c-a-b-c.'

Coordination Number and Number of Atoms Per Unit Cell

Coordination Number Body Centered Cubic

A unit cell is the smallest representation of an entire crystal. All crystal lattices are built of repeating unit cells. In a unit cell, an atom's coordination number is the number of atoms it is touching.

Coordination Number Of Simple Cubic System Is

The hexagonal closest packed (hcp) has a coordination number of 12 and contains 6 atoms per unit cell.
The face-centered cubic (fcc) has a coordination number of 12 and contains 4 atoms per unit cell.
The body-centered cubic (bcc) has a coordination number of 8 and contains 2 atoms per unit cell.
The simple cubic has a coordination number of 6 and contains 1 atom per unit cell.

Unit Cell	Coordination Number	# of Atoms Per Unit Cell	% space
Simple Unit Cell	6	1	52%
Body-Centered Cubic	8	2	68%
Face-centered Cubic	12	4	74.04%
Cubic Closest Packed	12	4	74.04%
Hexagonal Closest Packed	12	*6 (2) see note below**	74.04%

Note

*For the hexagonal close-packed structure the derivation is similar. Here the unit cell consist of three primitive unit cells is a hexagonal prism containing six atoms (if the particles in the crystal are atoms). Indeed, three are the atoms in the middle layer (inside the prism); in addition, for the top and bottom layers (on the bases of the prism), the central atom is shared with the adjacent cell, and each of the six atoms at the vertices is shared with other five adjacent cells. So the total number of atoms in the cell is 3 + (1/2)×2 + (1/6)×6×2 = 6, however this results in 2 per primitive unit cell.

References

Conway, J. H. and Sloane, N. J. A. Sphere Packings, Lattices, and Groups, 2nd ed. New York: Springer-Verlag, 1993.
Krishna, P. and Verma, A. R. Closed Packed Structures, Chester, UK: International Union of Crystallography, 1981.
Petrucci, Ralph H., William S. Harwood, F. Geoffrey Herring, and Jeffry D. Madura. 'Crystal Structures' General Chemistry: Principles & Modern Applications, ninth Edition. New Jersey: Pearson Education, Inc., 2007. 501-508.

Contributors and Attributions

How To Calculate Coordination Number Of Simple Cubic

Brittanie Harbick, Laura Suh, Jenny Fong